Orbital Mechanics for Dummies - Orbital Energy

Now we're going to consider the energy in an orbit, and we'll consider two forms of energy: kinetic energy from the speed of motion around the central body; and gravitational potential energy by virtue of the forces in a gravitational field, (which we'll call gravitational  energy for short).

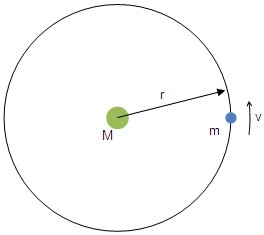
First we need to consider a reference point.  Here on Earth, it is tempting to consider being at rest on the ground to be the zero orbital energy point.  But that means for flight between different planets we have different references to consider and that won't do.  The solution is to consider the reference point to be stationary at an infinite distance from the central body, where the gravitational field is zero.  Then we can use this reference for flight between as many different bodies as we wish.

Kinetic energy is easy, and from high school physics I remember it is given by

E_k = ½mV²

But from our equation [1] we found that in a circular orbit,

V_C² = GM/r

So in such an orbit,

E_k = ½GMm/r = GMm/2r   [2]

This is an important equation and we'll call it [2].  Just take a moment to consider what this means: the kinetic energy of a body in circular orbit is proportional to the masses of the bodies, and inversely proportional to the distance between them.  So as the mass of either body increases, so the kinetic energy increases.  As the distance increases, so the kinetic energy decreases.  This fits with equation [1] which told us that as distance increases, so orbital speed decreases.

Now gravitational energy is a little harder to understand.  It is the work done by gravity to bring a body from infinite distance to a radius r from the central body.  At each step along the way, the gravity from the central body applies a force of GMm/r².  At each step dr along the way from infinity to r, the work done is the force GMm/r² multiplied by the distance dr.  And we add all those steps together by integrating the force from infinity to r as follows:

If calculus puts you off, feel free to take my word for it and skip to equation [3]

E_g = ∫_∞^r GMm/r² dr 

=  GMm  ∫_∞^r 1/r²

= GMm  [-1/r]_∞^r

= -GMm  [  (1/r)  - (1/∞) ]

= -GMm  [  (1/r)  - 0 ]

E_g = -GMm/r     [3]     <= equation [3]

This too is an important equation and we'll call it [3].  Just take a moment to consider what this means: the gravitational energy of a body in circular orbit is proportional to the masses of the bodies, and inversely proportional to the distance between them.  But it has a minus sign, I hear you say.  Yes indeed, and that means that gravitational energy increases (becomes less negative) as an object is lifted away from the central body.  That fits with the idea that energy is required to do work in lifting the object.  It also has some convenient consequences for orbital mechanics which we'll see later on.

Now that was the hardest piece of maths we'll do in this series, and there is no further calculus at all!  Whether you followed the integration or skipped it, please go back and make sure you understand these two energies because they lay the foundation for truly understanding orbits, and all kinds of interplanetary travel.

To wrap up orbital energy, we'll add kinetic energy and gravitational energy together into a single value.

E_o = E_k + E_g

= GMm/2r - GMm/r

E_o = -GMm/2r     [4]

Notice that orbital energy is negative, and increases in value as radius increases.  It's as though this energy represents how far the body is located down into the gravity well of the central body.  And if you imagine raising this energy past zero to a positive value as representing the orbiting body climbing out of the gravity well, then this will lead nicely on to the concept of escape velocity which we'll cover next time.

That's enough for this post.  Next time we'll consider how energy oscillates between kinetic and gravitational energies in elliptical orbits, and look at escape velocity.

Orbital Mechanics for Dummies - Kepler's Third Law

This post is an aside.  Here I show how to prove Kepler's third law of planetary motion from what we have done so far.  Nothing which follows depends on it, and if you choose to skip this post then you will lose nothing of substance from the series on Orbital Mechanics.

Kepler's third law states that

the square of the period of a planetary orbit is proportional to the cube of its distance from the Sun.

In one orbit, the orbiting body travels a distance equal to the circumference of the circle with radius r.  This distance is 2πr.  So the time taken to travel this distance, or the period of the orbit in seconds, P, is given by

P = 2πr / V_C

Taking the square of both sides gives

P² = 4π²r² / V_C²

Now from our last post we know that

V_C² = GM/r

Putting this into the equation for the period squared gives

P² = 4π²r² / (GM/r)

which simplifies to

P² = 4π²r³ / GM

Now if this equation is applied to planets orbiting the Sun, then the square of period of the orbit, P², is directly proportional to the cube of the distance from the Sun, r³.  So we have proved Kepler's third law.

Orbital Mechanics for Dummies - Circular Orbits

Last time we looked at the kinds of basic orbits, and gave a description of how the orbiting body moves in each.  Now we will dip our toe in the maths water.

The first step is to define the parameters of the orbit, according to the following diagram.

M is the mass of the central body, in kilograms (kg).
m is the mass of the orbiting body, in kilograms (kg).
r is the orbital radius between the centres of the bodies, in metres (m).
v is the speed at which the orbiting body moves around the central body, in metres per second (m/s).

There are a few other parameters we'll use along the way:

G is Newton's gravitational constant, and is equal to 6.67 x10^-11 m³/kg/s².

V_C is the speed of a body moving in a perfect circular orbit, in metres per second (m/s).

V_E is the speed of a body moving just fast enough to escape from orbit, in metres per second (m/s).

A is an acceleration, expressed as a change in speed per second (m/s²).

From the previous post we know that for a circular orbit the orbiting body moves under centripetal acceleration which is provided by the gravity field of the central body.  So we need formulae for both centripetal acceleration and gravitational acceleration.

From high school physics we recall that centripetal acceleration is given by

A_C = V²/r

and gravitational acceleration is given by

A_G = GM/r²

Now since centripetal acceleration is provided by gravitational acceleration, A_C = A_G so

V²/r = GM/r²

and because we can eliminate the /r on each side, and in a circular orbit V = V_C

V_C² = GM/r  [1]

There we have it, our first equation for orbital mechanics.  Wasn't so hard, was it?   It's such an important equation that I've labelled it [1] so we can refer to it again.  What it tells us is that the square of the orbital speed is proportional to the mass of the central body, and inversely proportional to the orbital radius.  The constant of proportionality is Newton's gravitational constant.

Example

To try this out, let's work out how fast the International Space Station (ISS) travels around the Earth.  As I remember, it's around 17,500mph.  But can our equation [1] confirm that?

The mass of the Earth is 5.97 x10²⁴ kg.

The radius of the Earth is 6.37 x10⁶ m.

The ISS orbits at 350km above the surface, so the orbital radius is 6.72 x10⁶ m.

V_C² = GM/r = 6.67 x10^-11 x 5.97 x10²⁴ / 6.72 x10⁶ = 59.3 x10⁶.

Taking the square root gives

V_C =7,700 m/s.

Since there are 1,610 metres in a mile, and 3,600 seconds in an hour,

Orbital Speed = 7,700 x 3,600 / 1,610 = 17,200 mph.

That works for me!  Next time we'll take on the giant of orbital mechanics and prove Kepler's third law.

Orbital Mechanics for Dummies - Orbital Basics

I’m going to deal with very simple situations, as these make both understanding and the maths easier, and most real situations can be usefully approximated by these simple cases.  The central body is vastly more massive than the orbiting body, so we can consider the central body to be fixed, and just work on the orbiting body.

The simplest orbit is perfectly circular.  The orbiting body moves in a circle at just the right speed so that its centrifugal force is precisely balanced by the gravitational force between the bodies.  We will call that speed V_C.

Now physics purists will tell us that centrifugal force is an illusion, so to satisfy them we’ll consider acceleration rather than force and describe the orbit as the path where centripetal acceleration is provided by the gravity of the central body.  We’ll do the maths for this in the next post.  For now, here’s the circular orbit as a diagram.

A variation of this orbit is the elliptical orbit.  If at point A the orbiting body accelerates by a small amount, (less than 41% of V_C), then as it proceeds around the central body its extra speed will cause it to climb away from the circular orbit path.  As it climbs against the force of gravity from the central body, the orbiting body decelerates.  It reaches its furthest point at B, directly opposite A, and begins to accelerate back towards the original point A once again.

 

Point A, the closest to the central body, is called periapsis.  Point B, the furthest from the central body, is called apoapsis.

When the orbiting body is at point B, it is travelling more slowly than the circular orbit speed, V_C’, at that radius from the central body.  Now if the orbiting body accelerates again at point B, to V_C’, it will follow a new circular orbit passing through B.

 

This kind of manoeuvre, where an elliptical transfer orbit is used to change between orbits of different radii, is used frequently in space flight.  Some satellites are put into geostationary orbits, where they orbit once in 24 hours and appear stationary over particular points on the equator as the Earth rotates.  When a geostationary satellite is launched, it is inserted into a transfer orbit with apoapsis at the geostationary radius.  Then a dedicated engine is fired at apoapsis to accelerate and achieve a circular geostationary orbit.  A variation of this kind of transfer orbit was used by Apollo spacecraft to reach the Moon.

Finally, if the orbiting body accelerates by 41% of V_C or more, then this speed causes it to climb away as before, but this time it is travelling fast enough that the gravity field from the central body reduces more quickly than the body decelerates, and the body continues climbing indefinitely.  The body has exceeded the escape velocity, V_E, has escaped the gravity field and will not return.

These are the basic principles to grasp for now.  Next time we will look at some simple maths to describe these different cases.

Orbital Mechanics for Dummies - Introduction

I have been following the Dawn mission to asteroid Vesta with keen interest.  The mission team is relying on simulation data because for various reasons accurate measurements of the probe's position are only available occasionally.  The probe is expected to achieve orbital insertion around Vesta today, but without accurate data not available until tomorrow, we can only speculate on whether orbit has been achieved.

For hour-by-hour data, the team relies on the MYSTIC simulator.  This gives vital data including Dawn's speed in relation to Vesta, and its range to the asteroid.

I'm no rocket scientist, but I do have basic skills in physics and maths, and a keen interest in space flight.  A number of years ago, I worked out my own very basic system for understanding orbital mechanics, including the basic equations for simple orbits, from first principles.  I used these, with the simulation data, to show that Dawn appeared to have achieved orbital insertion around Vesta earlier this morning.

I have discussed my calculations with others interested in this mission.  It occurs to me that there may be other keen amateurs out there interested in understanding the basics of orbital mechanics.  So it is my intention to publish a series of posts on the subject.

It won't be for everyone, by a long way.  But if you have a good grasp of high school physics and maths, you should be able to follow along.  My own maths ability reached its limit shortly after high school level, so I haven't included anything too demanding!  It also won't satisfy real rocket scientists, who use levels of calculus way beyond me and can tell you all the fundamentals I've missed out.  However, if my basic version is flawed rather than just simplistic, then I'd really like to be corrected so please do get in touch!

If you're interested, keep an eye out for my following posts which detail my Orbital Mechanics for Dummies...

By the way, the title is not intended to patronize.  I consider myself to be a mathematical dummy, at least compared to the abilities of my peers when I was studying.  My aim is to use my limited skills to understand how orbits work, and how to work out their parameters.  There are plenty of more complete works on the subject, but this is intended to be an easy entry.